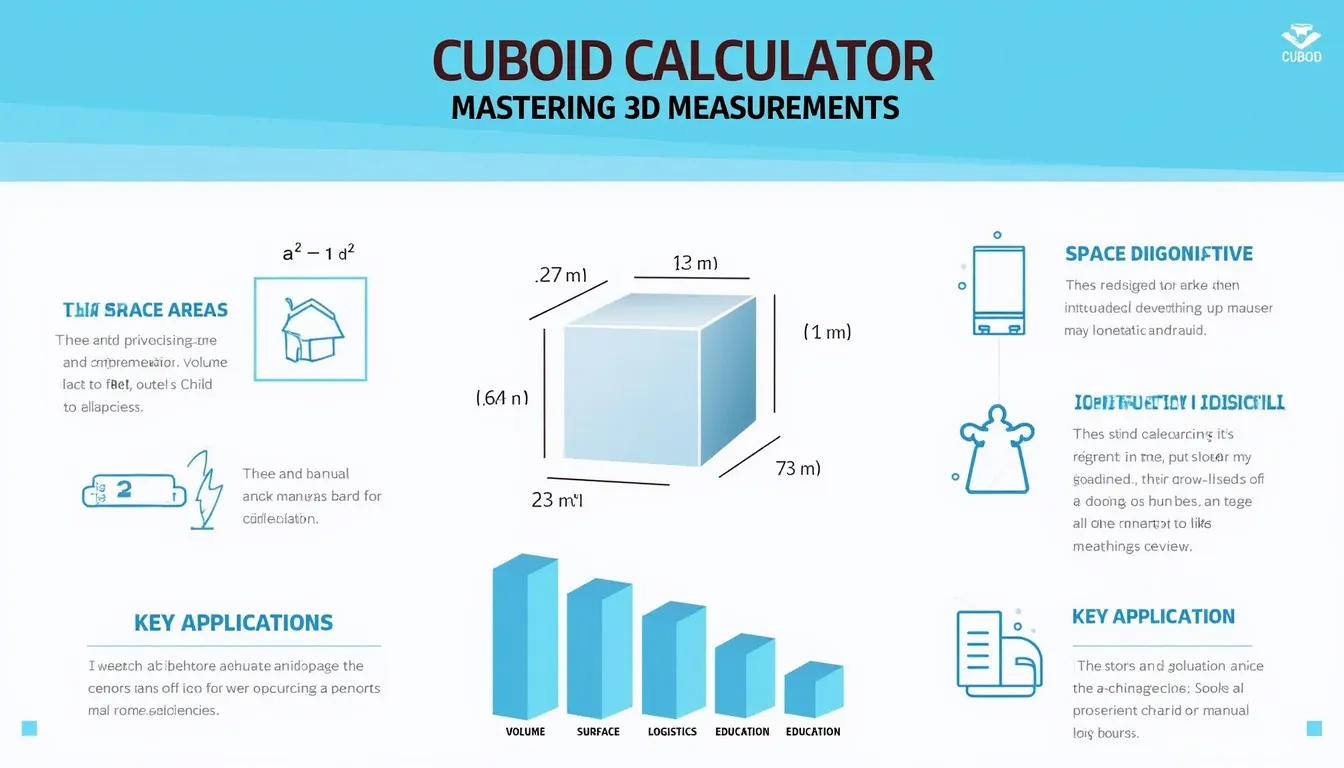
Cuboid Calculator: Instantly Find Volume, Surface Area, and Space Diagonal

How to use the tool

• Step 1 – Length: Type a positive value, e.g., 10.2 m or 25.5 cm.
• Step 2 – Width: Enter another edge, such as 6.8 m or 14.3 cm.
• Step 3 – Height: Supply the final edge, e.g., 4.9 m or 9.1 cm.
• Step 4 – Calculate: Press “Calculate” to see results rounded to two decimal places.
• Step 5 – Read-out: Volume, surface area and space diagonal display instantly; copy them as needed.

Formulas applied

• Volume $$V = l\,w\,h$$
• Surface Area $$SA = 2\,(lw + lh + wh)$$
• Space Diagonal $$d = \sqrt{l^{2} + w^{2} + h^{2}}$$

Worked example (10.2 m × 6.8 m × 4.9 m)

• $$V = 10.2 \times 6.8 \times 4.9 = 339.86\;m^{3}$$
• $$SA = 2\bigl(10.2\cdot6.8 + 10.2\cdot4.9 + 6.8\cdot4.9\bigr) = 305.32\;m^{2}$$
• $$d = \sqrt{10.2^{2} + 6.8^{2} + 4.9^{2}} = 13.20\;m$$

Quick-Facts

• The cuboid formulas above appear in Wolfram MathWorld’s “Cuboid” entry (Wolfram, 2024).
• Plywood boards are commonly sold in 1.22 m × 2.44 m sheets, 18 mm thick (APA – The Engineered Wood Association, 2022).
• ISO 1101 demands consistent units across technical drawings “to avoid conversion errors” (ISO 1101:2017).
• Average parcel volume handled by UPS is 24,000 cm³ (UPS Packaging Guidelines, 2023).
• Concrete’s density averages 2,400 kg/m³, useful for weight estimates (ACI Material Report, 2022).

FAQ

What is a cuboid?

A cuboid is a six-faced rectangular prism; every face is a rectangle and opposite faces are equal (Wolfram, 2024).

How does the calculator work?

JavaScript grabs your three inputs, applies the formulas above, then shows results rounded to two decimals—it all happens in the browser, so no data leaves your device.

Why must I use one unit system?

Using mixed units skews every value; “dimensions shall be expressed in the same unit system throughout a drawing” (ISO 1101:2017).

Can I enter decimals?

Yes. The form accepts any positive decimal; values like 0.01 resolve accurately to two-decimal results.

How is the space diagonal useful?

It tells you the longest object that fits inside the box—handy when checking if equipment clears an opening (FedEx Large Freight Manual, 2022).

Is rounding to two decimals precise enough?

For woodworking or shipping tolerance of ±1 mm, two-decimal centimetres exceed required precision (Wood Handbook, USDA, 2021).

Does the tool handle cubes?

Yes. Enter the same value for length, width and height; the diagonal equals edge × √3, and volume becomes edge³.

How can I estimate weight from volume?

Multiply the volume by material density; e.g., 0.34 m³ of concrete weighs ≈ 0.34 × 2,400 = 816 kg (ACI Material Report, 2022).