Variance Calculator: Easy-to-Use Statistical Tool for Data Analysis
Unlock the power of data analysis with our user-friendly Variance Calculator. Simplify complex statistical calculations, gain valuable insights, and make informed decisions across various fields. From academic research to financial analysis, discover how variance can revolutionize your approach to data. Ready to elevate your analytical skills? Explore now!
Variance Calculator
Results:
★ Add to Home Screen
×
Follow these steps:
Tap the Share button at the bottom of the browser
Scroll down and tap “Add to Home Screen”
Tap “Add” to confirm
Follow these steps:
Tap the menu icon (⋮) in the top right
Tap “Add to Home screen”
Tap “Add” to confirm
Follow these steps:
Click the menu icon (⋮) in the top right
Click “Bookmarks”
Click “Bookmark this tab” or press Ctrl+D (Cmd+D on Mac)
Is this tool helpful?
Thanks for your feedback!
Welcome to our comprehensive Variance Calculator, a powerful tool designed to help you analyze the spread of your data sets quickly and accurately. Whether you’re a student, researcher, or professional working with statistics, this calculator simplifies the process of computing variance, providing valuable insights into your data’s distribution.
How to Use the Variance Calculator Effectively
Using our Variance Calculator is straightforward and user-friendly. Follow these simple steps to get accurate results:
Enter your observations in the input field labeled “Observations”.
Separate each number with a space (e.g., 1 2 3 4 5).
Click the “Calculate” button to process your data.
View the results, which include both the variance and mean of your data set.
It’s important to note that you need to enter at least two numbers for the calculator to work correctly. If you enter fewer than two numbers or include non-numeric values, the calculator will prompt you to correct your input.
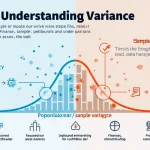
Understanding Variance: Definition and Purpose
Variance is a fundamental concept in statistics that measures the spread or dispersion of a set of numbers from their average (mean) value. It provides crucial information about how far a set of numbers are spread out from their average.
Time-saving: Quickly compute variance without manual calculations.
Accuracy: Eliminate human error in complex mathematical operations.
Convenience: Access the tool anytime, anywhere with an internet connection.
User-friendly: Simple interface suitable for users of all skill levels.
Comprehensive results: Get both variance and mean in one calculation.
Versatility: Applicable to various fields including finance, science, and social studies.
Addressing User Needs and Solving Problems
Our Variance Calculator addresses several common challenges faced by individuals working with statistical data:
1. Simplifying Complex Calculations
Calculating variance manually can be time-consuming and prone to errors, especially with large data sets. Our calculator automates this process, providing instant and accurate results.
2. Enhancing Data Analysis
By quickly providing variance values, the calculator enables users to gain deeper insights into their data’s distribution, facilitating more informed decision-making.
3. Improving Research Quality
For researchers and students, accurate variance calculations are crucial for statistical analysis. Our tool ensures precise results, contributing to the overall quality of research and academic work.
4. Supporting Financial Analysis
In finance, variance is essential for assessing risk and volatility. Our calculator aids financial analysts in quickly evaluating investment portfolios and market trends.
5. Facilitating Quality Control
Manufacturing and production processes often rely on variance to monitor product consistency. This calculator helps quality control teams efficiently analyze production data.
Practical Applications and Use Cases
The Variance Calculator finds applications across various fields. Here are some practical examples:
1. Academic Research
A psychology researcher studying reaction times across different age groups can use the calculator to determine the variance in each group’s responses, helping to identify age-related differences in cognitive processing speed.
2. Financial Analysis
An investment analyst can use the calculator to compute the variance of stock returns, providing insights into the volatility and risk associated with different securities.
3. Manufacturing Quality Control
A production manager in a food processing plant can use the calculator to analyze the variance in the weight of packaged products, ensuring consistency and compliance with regulatory standards.
4. Environmental Studies
An environmental scientist studying climate change can use the calculator to analyze the variance in temperature readings over time, helping to identify significant climate trends.
5. Sports Analytics
A sports analyst can use the calculator to compute the variance in player performance statistics, providing insights into consistency and reliability across different games or seasons.
Understanding the Results
When you use our Variance Calculator, you’ll receive two key pieces of information:
1. Variance
This value represents the average squared deviation from the mean. A higher variance indicates that the data points are more spread out, while a lower variance suggests they are clustered closer to the mean.
2. Mean
The mean is the average of all the numbers in your data set. It’s calculated by summing all the values and dividing by the number of values.
Interpreting these results together can provide valuable insights into your data’s characteristics and distribution.
Tips for Effective Data Analysis
To make the most of our Variance Calculator and enhance your data analysis, consider the following tips:
Data Preparation: Ensure your data is clean and properly formatted before input.
Sample Size: Use larger sample sizes when possible for more reliable variance estimates.
Context Matters: Always interpret variance in the context of your specific field or study.
Combine with Other Measures: Use variance alongside other statistical measures like mean, median, and standard deviation for a comprehensive analysis.
Visualize Your Data: Consider creating visual representations of your data to complement the variance calculations.
Frequently Asked Questions (FAQ)
Q1: What is the difference between variance and standard deviation?
A1: Variance measures the average squared deviation from the mean, while standard deviation is the square root of the variance. Standard deviation is often preferred as it’s in the same units as the original data.
Q2: Can I use this calculator for population variance?
A2: This calculator computes sample variance. For population variance, you would need to divide by n instead of (n-1) in the formula.
Q3: Why do we use (n-1) instead of n in the variance formula?
A3: Using (n-1) gives an unbiased estimate of the population variance when working with a sample. This is known as Bessel’s correction.
Q4: How does variance relate to data spread?
A4: A larger variance indicates that the data points are more spread out from the mean, while a smaller variance suggests they are clustered closer to the mean.
Q5: Can variance be negative?
A5: No, variance is always non-negative because it involves squared differences.
Q6: How accurate is this Variance Calculator?
A6: Our calculator uses standard mathematical formulas and is designed to provide accurate results. However, as with any digital tool, it’s important to note that we can’t guarantee that the webtool or results from our webtool are always correct, complete, or reliable. Our content and tools might have mistakes, biases, or inconsistencies.
Conclusion: Harness the Power of Variance Analysis
Our Variance Calculator is a powerful tool that simplifies complex statistical calculations, enabling you to gain valuable insights into your data’s distribution quickly and accurately. By providing both variance and mean results, it offers a comprehensive view of your data’s characteristics.
Whether you’re a student tackling statistical problems, a researcher analyzing experimental data, or a professional making data-driven decisions, this calculator can significantly enhance your analytical capabilities. It saves time, ensures accuracy, and allows you to focus on interpreting results rather than getting bogged down in manual calculations.
Remember, while variance is a crucial statistical measure, it’s most powerful when used in conjunction with other analytical tools and within the specific context of your field or study. Always consider the broader picture when interpreting your results.
We encourage you to make full use of this Variance Calculator in your data analysis endeavors. By understanding and leveraging the power of variance, you can uncover meaningful patterns, make more informed decisions, and drive your projects to success.
Start using our Variance Calculator today and take your data analysis to the next level!
Important Disclaimer
The calculations, results, and content provided by our tools are not guaranteed to be accurate, complete, or reliable. Users are responsible for verifying and interpreting the results. Our content and tools may contain errors, biases, or inconsistencies. We reserve the right to save inputs and outputs from our tools for the purposes of error debugging, bias identification, and performance improvement. External companies providing AI models used in our tools may also save and process data in accordance with their own policies. By using our tools, you consent to this data collection and processing. We reserve the right to limit the usage of our tools based on current usability factors. By using our tools, you acknowledge that you have read, understood, and agreed to this disclaimer. You accept the inherent risks and limitations associated with the use of our tools and services.
