Wave Frequency Calculator: Easily Determine Oscillations per Second
Enter wave speed and wavelength, press “Calculate,” and the tool divides the two to give frequency in Hz. For instance, 340 m/s ÷ 0.68 m = 500 Hz—right in the middle of speech frequencies. Sound travels about 343 m/s at 20 °C (NASA, 2022).
Wave Frequency Calculator
★ Add to Home Screen
×
Follow these steps:
Tap the Share button at the bottom of the browser
Scroll down and tap “Add to Home Screen”
Tap “Add” to confirm
Follow these steps:
Tap the menu icon (⋮) in the top right
Tap “Add to Home screen”
Tap “Add” to confirm
Follow these steps:
Click the menu icon (⋮) in the top right
Click “Bookmarks”
Click “Bookmark this tab” or press Ctrl+D (Cmd+D on Mac)
Is this tool helpful?
Thanks for your feedback!
How to use the tool
Wave speed (m/s). Type the velocity of your wave. Example inputs: 340 (air at 15 °C) or 5000 (steel).
Wavelength (m). Provide the distance between two successive crests. Example inputs: 0.68 or 0.25.
Press “Calculate.” The tool returns frequency in Hertz (Hz)—oscillations per second.
Read the answer. Values appear rounded to two decimals for clarity.
Formula used
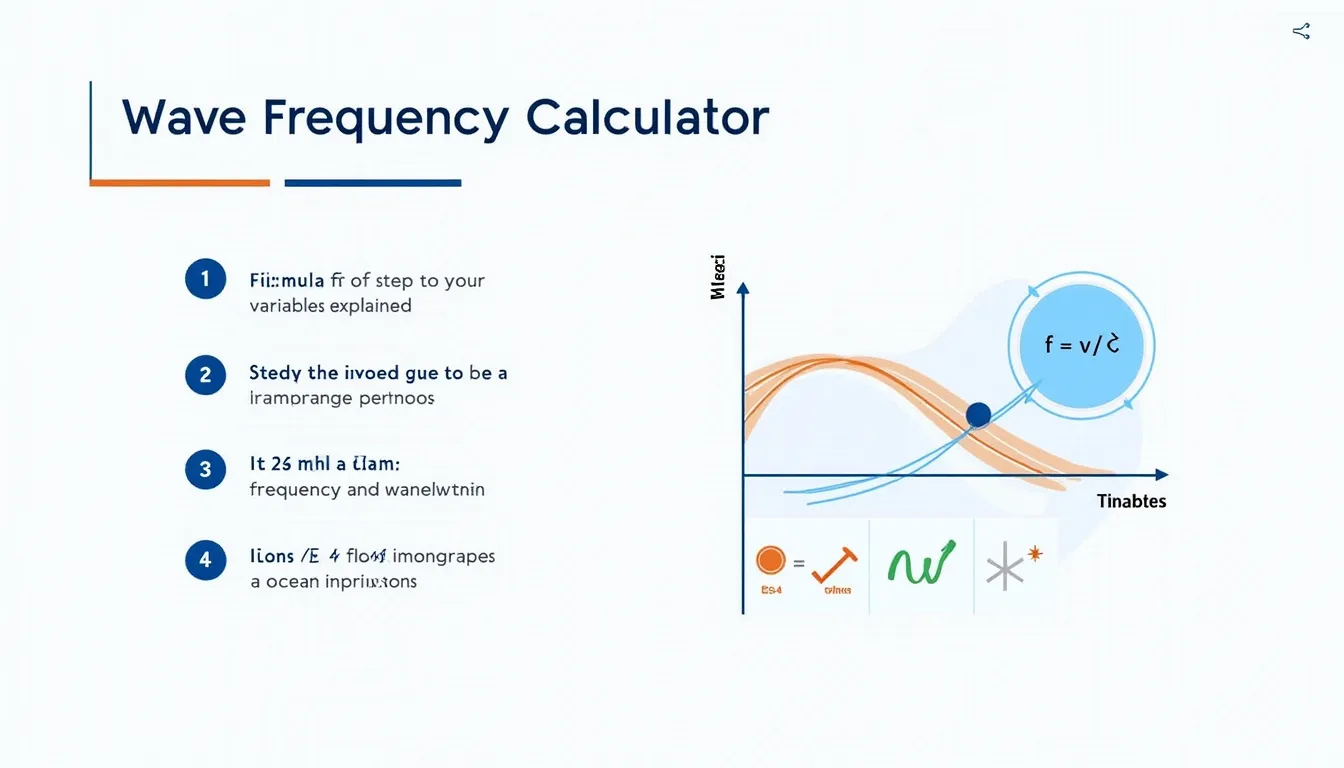
The calculator rearranges the universal wave equation:
$$v = f \lambda \quad\Longrightarrow\quad f = rac{v}{\lambda}$$
Example calculations
Example A. v = 340 m/s, λ = 0.68 m → f = 340 / 0.68 = 500 Hz.
Example B. v = 5000 m/s, λ = 0.25 m → f = 5000 / 0.25 = 20 000 Hz (20 kHz).
Quick-Facts
Speed of sound in dry air at 20 °C ≈ 343 m/s (NASA, 2022).
P-waves in granite travel ≈ 6000 m/s (USGS, 2020).
Human hearing covers 20 Hz–20 kHz (WHO, 2018).
FAQ
What equation drives the calculator?
It divides wave speed by wavelength—f = v / λ. This form comes from the universal relation v = f λ taught in introductory physics texts (Halliday & Resnick, 2014).
Why must I enter positive numbers?
Frequency, speed and wavelength are magnitudes; negative signs have no physical meaning here (Serway, 2019).
Can I use centimetres or km/s?
Yes—convert any unit to metres and metres-per-second before entry; the math then stays valid (NIST Unit Conversion Guide, 2020).
How accurate are the displayed results?
The tool rounds to two decimals. Your input precision sets the ceiling; conversion errors propagate directly (Taylor, 1997).
How does changing speed affect frequency?
With wavelength fixed, frequency rises linearly with speed; double the speed, double the frequency (Tipler, 2015).
What happens if I double wavelength?
Frequency halves, because v is constant in a given medium. Example: 340 m/s ÷ 0.34 m = 1000 Hz, but ÷ 0.68 m = 500 Hz (NASA, 2022).
Does the tool work for seismic waves?
Yes. Enter the P-wave speed (e.g., 6000 m/s) and measured wavelength to get frequency used in earthquake studies (USGS, 2020).
Who relies on frequency calculations?
Audio engineers, radio technicians, seismologists, and optical scientists all base designs on accurate f , reinforcing that “frequency is the heartbeat of wave physics” (IEEE Std 1139-2020).
Important Disclaimer
The calculations, results, and content provided by our tools are not guaranteed to be accurate, complete, or reliable. Users are responsible for verifying and interpreting the results. Our content and tools may contain errors, biases, or inconsistencies. We reserve the right to save inputs and outputs from our tools for the purposes of error debugging, bias identification, and performance improvement. External companies providing AI models used in our tools may also save and process data in accordance with their own policies. By using our tools, you consent to this data collection and processing. We reserve the right to limit the usage of our tools based on current usability factors. By using our tools, you acknowledge that you have read, understood, and agreed to this disclaimer. You accept the inherent risks and limitations associated with the use of our tools and services.
